Skip to main content

Exercises 2.6 Practice Problems

Exercise Group.

For each of the following functions, evaluate at the given input.

  • \(\displaystyle f(3)\)

  • \(\displaystyle f(4)\)

  • \(\displaystyle f(-1)\)

  • \(\displaystyle f\left(\frac{3}{4}\right)\)

1.

\(f(x)=3-8x\)

Answer.

  • \(\displaystyle f(3)=-21\)

  • \(\displaystyle f(4)=-29\)

  • \(\displaystyle f(-1)=11\)

  • \(\displaystyle f\left(\frac{3}{4}\right)=-3\)

2.

\(f(x)=6x-4\)

Answer.

  • \(\displaystyle f(3)=14\)

  • \(\displaystyle f(4)=20\)

  • \(\displaystyle f(-1)=-10\)

  • \(\displaystyle f\left(\frac{3}{4}\right)=\left(\frac{1}{2}\right)\)

3.

\(f(x)=26\)

Answer.

  • \(\displaystyle f(3)=26\)

  • \(\displaystyle f(4)=26\)

  • \(\displaystyle f(-1)=26\)

  • \(\displaystyle f\left(\frac{3}{4}\right)=26\)

4.

\(f(x)=-x+6\)

Answer.

  • \(\displaystyle f(3)=3\)

  • \(\displaystyle f(4)=2\)

  • \(\displaystyle f(-1)=7\)

  • \(\displaystyle f\left(\frac{3}{4}\right)=5 \frac{1}{4} \)

5.

\(f(x)=2x\)

Answer.

  • \(\displaystyle f(3)=6\)

  • \(\displaystyle f(4)=8\)

  • \(\displaystyle f(-1)=-2\)

  • \(\displaystyle f\left(\frac{3}{4}\right)= \frac{3}{2}\)

6.

\(f(x)=-\left(\frac{2}{3}\right)x + 1\)

Answer.

  • \(\displaystyle f(3)=-1\)

  • \(\displaystyle f(4)=-\frac{5}{3}\)

  • \(\displaystyle f(-1)=\frac{5}{3}\)

  • \(\displaystyle f\left(\frac{3}{4}\right)=\frac{1}{2} \)

Exercise Group.

For each of the following functions, evaluate at the given input.

  • \(\displaystyle g(c)\)

  • \(\displaystyle g(2e)\)

  • \(\displaystyle g(-k)\)

  • \(\displaystyle g\left(\frac{1}{2}h\right)\)

7.

\(g(x)=2-4x\)

Answer.

  • \(\displaystyle g(c)=2-4c\)

  • \(\displaystyle g(2e)=2-8e\)

  • \(\displaystyle g(-k)=2+4k\)

  • \(\displaystyle g\left(\frac{1}{2}h\right)=2-2h\)

8.

\(f(x)=3x-3\)

Answer.

  • \(\displaystyle g(c)=3c-3\)

  • \(\displaystyle g(2e)=6e-3\)

  • \(\displaystyle g(-k)=-3k-3\)

  • \(\displaystyle g\left(\frac{1}{2}h\right)=\frac{3}{2}h-3\)

9.

\(g(x)=12\)

Answer.

  • \(\displaystyle g(c)=12\)

  • \(\displaystyle g(2e)=12\)

  • \(\displaystyle g(-k)=12\)

  • \(\displaystyle g\left(\frac{1}{2}h\right)=12\)

10.

\(g(x)=x-1\)

Answer.

  • \(\displaystyle g(c)=c-1\)

  • \(\displaystyle g(2e)=2e-1\)

  • \(\displaystyle g(-k)=-k-1\)

  • \(\displaystyle g\left(\frac{1}{2}h\right)=\frac{1}{2}h-1\)

11.

\(g(x)=x\)

Answer.

  • \(\displaystyle g(c)=c\)

  • \(\displaystyle g(2e)=2e\)

  • \(\displaystyle g(-k)=-k\)

  • \(\displaystyle g\left(\frac{1}{2}h\right)=\frac{1}{2}h\)

12.

\(g(x)=-\frac{1}{4}x + 2\)

Answer.

  • \(\displaystyle g(c)=-\frac{1}{4}c+2\)

  • \(\displaystyle g(2e)=-\frac{1}{2}e+2\)

  • \(\displaystyle g(-k)=\frac{1}{4}k+2\)

  • \(\displaystyle g\left(\frac{1}{2}h\right)=\frac{1}{8}h+2\)

Exercise Group.

For each of the following functions, evaluate the given input.

  • \(\displaystyle f(x) = 2x+3\)

  • \(\displaystyle g(x) = 10x\)

  • \(\displaystyle h(x) = 0\)

  • \(\displaystyle y(x) = \frac{1}{5}x\)

13.

Evaluate all four of the above functions given \(x=1 \text{.}\)

Answer.

  • \(\displaystyle f(1)=5\)

  • \(\displaystyle g(1)=10\)

  • \(\displaystyle h(1)=0\)

  • \(\displaystyle y(1)=\frac{1}{5}\)

14.

Evaluate all four of the above functions given \(x=a \text{.}\)

Answer.

  • \(\displaystyle f(a)=2a+3\)

  • \(\displaystyle g(a)=10a\)

  • \(\displaystyle h(a)=0\)

  • \(\displaystyle y(a)=\frac{1}{5}a\)

15.

Evaluate all four of the above functions given \(x=0 \text{.}\)

Answer.

  • \(\displaystyle f(0)=3\)

  • \(\displaystyle g(0)=0\)

  • \(\displaystyle h(0)=0\)

  • \(\displaystyle y(0)=0\)

16.

Evaluate all four of the above functions given \(x=2z \text{.}\)

Answer.

  • \(\displaystyle f(2z)=4z+3\)

  • \(\displaystyle g(2z)=20z\)

  • \(\displaystyle h(2z)=0\)

  • \(\displaystyle y(2z)=\frac{2}{5}z\)

17.

Evaluate all four of the above functions given \(x=\frac{1}{2} \text{.}\)

Answer.

  • \(\displaystyle f\left(\frac{1}{2}\right)=4\)

  • \(\displaystyle g\left(\frac{1}{2}\right)=5\)

  • \(\displaystyle h\left(\frac{1}{2}\right)=0\)

  • \(\displaystyle y\left(\frac{1}{2}\right)=\frac{1}{10}\)

18.

Evaluate all four of the above functions given \(x=b+5 \text{.}\)

Answer.

  • \(\displaystyle f(b+5)=2b+13\)

  • \(\displaystyle g(b+5)=10b + 50\)

  • \(\displaystyle h(b+5)=0\)

  • \(\displaystyle y(b+5)=\frac{1}{5}b + 1\)

19.

Suppose \(a(t)= \frac{1}{19}\text{.}\)

  1. Evaluate \(a(1)\text{.}\)

  2. Evaluate \(a(b+c)\text{.}\)

  3. Evaluate \(a(9)\text{.}\)

  4. Evaluate \(a(\frac{1}{19})\text{.}\)

Answer.

  1. \(\displaystyle a(1) = \frac{1}{19}\)

  2. \(\displaystyle a(b+c)) = \frac{1}{19}\)

  3. \(\displaystyle a(9) = \frac{1}{19}\)

  4. \(\displaystyle a\left(\frac{1}{19}\right) = \frac{1}{19}\)

20.

Suppose \(d(t)=\pi t\text{.}\)

  1. Evaluate \(d(0)\text{.}\)

  2. Evaluate \(d(a+h)\text{.}\)

  3. Evaluate \(d(x)\text{.}\)

  4. Evaluate \(d(\sqrt{x+3})\text{.}\)

Answer.

  1. \(\displaystyle d(0) = 0\)

  2. \(\displaystyle d(a+h) = \pi a + \pi h\)

  3. \(\displaystyle d(x) = \pi x\)

  4. \(\displaystyle d(\sqrt{x+3}) = \left( \pi \right) \left( \sqrt{x+3} \right)\)

21.

Suppose

\begin{equation*} g(x) = \begin{cases}\sqrt{x} \amp x \gt 2 \\ x^2 \amp -3 \lt x \le 2 \\ x+3 \amp x \le -3 \end{cases} \end{equation*}

We want to evaluate each of the following:

  1. \(\displaystyle g(0)\)

  2. \(\displaystyle g(-5)\)

  3. \(\displaystyle g(10)\)

  4. \(\displaystyle g(2)\)

Answer.

  1. \(\displaystyle g(0)=0\)

  2. \(\displaystyle g(-5)= -2\)

  3. \(\displaystyle g(10)= \sqrt{10}\)

  4. \(\displaystyle g(2) = 4\)

22.

Suppose

\begin{equation*} h(t) = \begin{cases}\frac{1}{2}t \amp t \ge 3 \\ t^3 \amp 0 \lt t \lt 3 \\ t+3 \amp t \le 0 \end{cases} \end{equation*}

We want to evaluate each of the following:

  1. \(\displaystyle h(1)\)

  2. \(\displaystyle h(3)\)

  3. \(\displaystyle h(0)\)

  4. \(\displaystyle h(-3)\)

Answer.

  1. \(\displaystyle h(1) = 1\)

  2. \(\displaystyle h(3) = \frac{3}{2}\)

  3. \(\displaystyle h(0) = 3\)

  4. \(\displaystyle h(-3) = 0\)

23.

Suppose

\begin{equation*} f(t) = \begin{cases}\frac{1}{3}t \amp t \ge 5 \\ \sqrt{t} \amp 1 \lt t \lt 5 \\ t \amp t \le 1 \end{cases} \end{equation*}

We want to evaluate each of the following:

  1. \(\displaystyle f(1)\)

  2. \(\displaystyle f(5)\)

  3. \(\displaystyle f(0)\)

  4. \(\displaystyle f\left(\frac{3}{2}\right)\)

Answer.

  1. \(\displaystyle f(1) = 1\)

  2. \(\displaystyle f(5) = \frac{5}{3}\)

  3. \(\displaystyle f(0) = 0\)

  4. \(\displaystyle f\left(\frac{3}{2}\right) = \sqrt{\frac{3}{2}}\)

24.

Suppose

\begin{equation*} f(x) = \begin{cases}\frac{1}{x} \amp x \gt 0 \\ x \amp x=0 \\ -\frac{1}{x} \amp x \lt 0 \end{cases} \end{equation*}

We want to evaluate each of the following:

  1. \(\displaystyle f(-1)\)

  2. \(\displaystyle f(0)\)

  3. \(\displaystyle f(2)\)

  4. \(\displaystyle f\left(\frac{1}{2}\right)\)

Answer.

  1. \(\displaystyle f(-1) = 1\)

  2. \(\displaystyle f(0) = 0\)

  3. \(\displaystyle f(2) = \frac{1}{2}\)

  4. \(\displaystyle f\left(\frac{1}{2}\right) = 2\)

25.

Suppose

\begin{equation*} f(x) = \begin{cases}\frac{1}{2} \amp x \gt 10 \\ 2x \amp x=10 \\ -1 \amp x \lt 10 \end{cases} \end{equation*}

We want to evaluate each of the following:

  1. \(\displaystyle f(-10)\)

  2. \(\displaystyle f(0)\)

  3. \(\displaystyle f(10)\)

  4. \(\displaystyle f(20)\)

Answer.

  1. \(\displaystyle f(-10)=-1\)

  2. \(\displaystyle f(0)=-1\)

  3. \(\displaystyle f(10)=20\)

  4. \(\displaystyle f(20)=\frac{1}{2}\)

26.

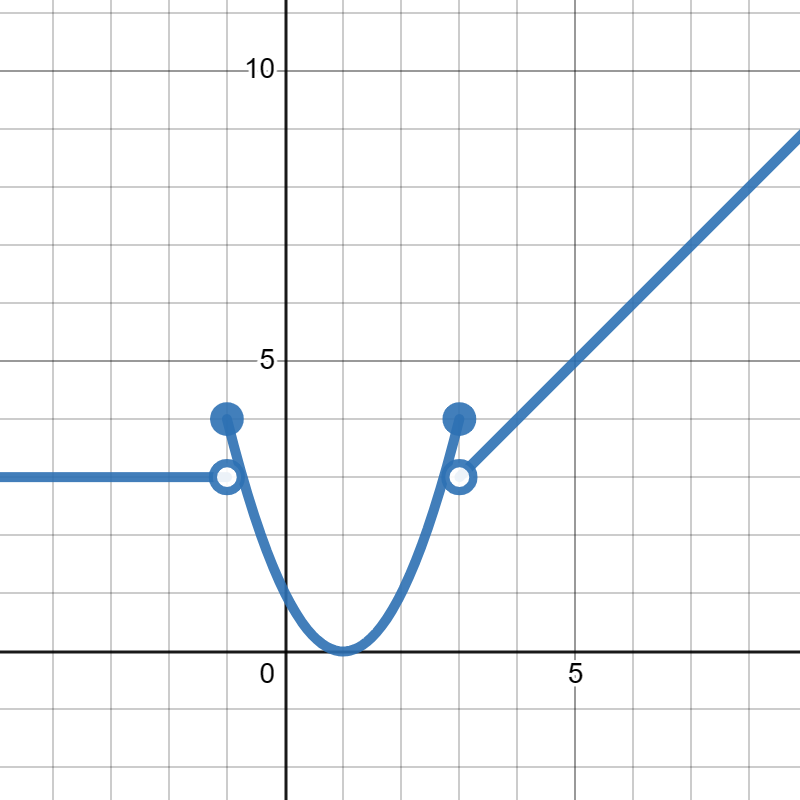
Suppose \(g(x)\) is given in the graph below:

Evaluate each of the following:

  1. \(\displaystyle g(-2)\)

  2. \(\displaystyle g(4)\)

  3. \(\displaystyle g(6)\)

  4. \(\displaystyle g(8)\)

  5. \(\displaystyle g(9)\)

Answer.

  1. \(\displaystyle g(-2)=-1\)

  2. \(\displaystyle g(4)=3\)

  3. \(\displaystyle g(6)=5\)

  4. \(\displaystyle g(8)=11\)

  5. \(\displaystyle g(9)=12\)

27.

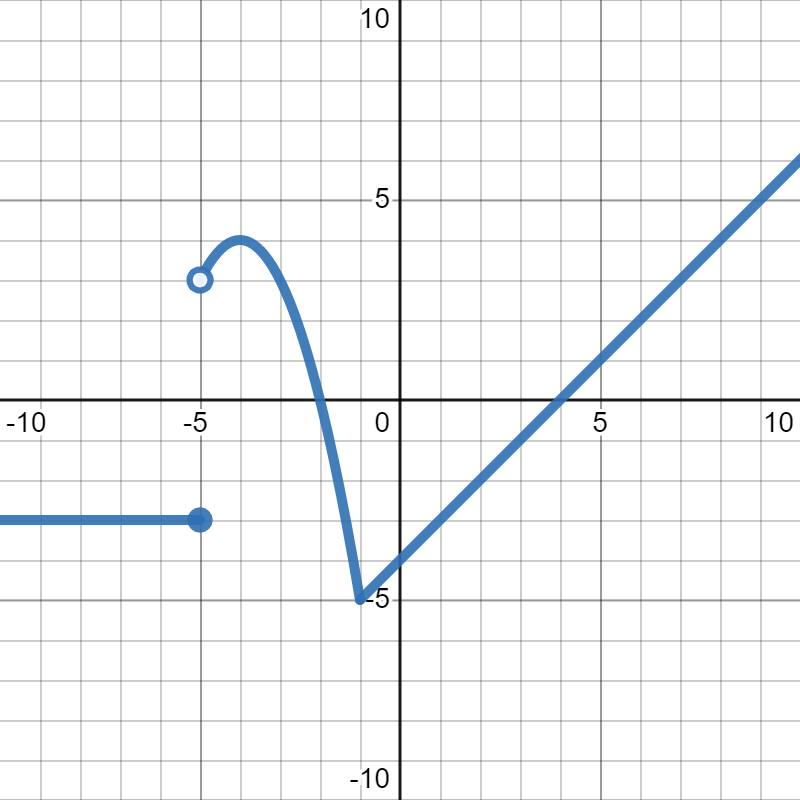
Suppose \(f(x)\) is given in the graph below:

Evaluate each of the following:

  1. \(\displaystyle f(-3)\)

  2. \(\displaystyle f(0)\)

  3. \(\displaystyle f(3)\)

  4. \(\displaystyle f(5)\)

  5. \(\displaystyle f(7)\)

Answer.

  1. \(\displaystyle f(-3)=9\)

  2. \(\displaystyle f(0)=0\)

  3. \(\displaystyle f(3)=9\)

  4. \(\displaystyle f(5)=13\)

  5. \(\displaystyle f(7)=17\)

28.

Suppose \(f(x)\) is given in the table below:

Table 2.35.
\(x\) \(f(x)\)
\(-3\) \(1\)
\(0\) \(2\)
\(3\) \(-7\)
\(6\) \(/pi\)
\(\frac{1}{3}\) \(4.31\)

Evaluate each of the following:

  1. \(\displaystyle f(-3)\)

  2. \(\displaystyle f(0)\)

  3. \(\displaystyle f(3)\)

  4. \(\displaystyle f(6)\)

  5. \(\displaystyle f(\frac{1}{3})\)

Answer.

  1. \(\displaystyle f(-3)=1\)

  2. \(\displaystyle f(0)=2\)

  3. \(\displaystyle f(3)=-7\)

  4. \(\displaystyle f(6)=\pi\)

  5. \(\displaystyle f(\frac{1}{3})=4.31\)

29.

Suppose \(f(t)\) is given in the table below:

Table 2.36.
\(t\) \(f(t)\)
\(0\) \(1\)
\(1\) \(2\)
\(2\) \(3\)
\(3\) \(4\)
\(4\) \(5\)

Evaluate each of the following:

  1. \(\displaystyle f(0)\)

  2. \(\displaystyle f(1)\)

  3. \(\displaystyle f(2)\)

  4. \(\displaystyle f(3)\)

  5. \(\displaystyle f(4)\)

Answer.

  1. \(\displaystyle f(0)=1\)

  2. \(\displaystyle f(1)=2\)

  3. \(\displaystyle f(2)=3\)

  4. \(\displaystyle f(3)=4\)

  5. \(\displaystyle f(4)=5\)

30.

Suppose \(f(x)\text{,}\) \(g(x)\text{,}\) and \(h(x)\) are given in the table below:

Table 2.37.
x f(x) g(x) h(x)
0 9 4 1
1 -2 5.5 -3
2 6 6 6
3 7 0 8
4 -1 8 -4

Evaluate each of the following:

  1. \(\displaystyle h(0)\)

  2. \(\displaystyle f(1)\)

  3. \(\displaystyle g(2)\)

  4. \(\displaystyle f(3)\)

  5. \(\displaystyle h(4)\)

Answer.

  1. \(\displaystyle h(0)=1\)

  2. \(\displaystyle f(1)=-2\)

  3. \(\displaystyle g(2)=6\)

  4. \(\displaystyle f(3)=7\)

  5. \(\displaystyle h(4)=-4\)

31.

Suppose \(m(x)\) is given in the table below:

Table 2.38.
x 1 3 5 7 9
m(x) 6 2 -1 -5 -8

Evaluate each of the following:

  1. \(\displaystyle m(5)\)

  2. \(\displaystyle m(1)\)

  3. \(\displaystyle m(9)\)

  4. \(\displaystyle m(3)\)

  5. \(\displaystyle m(7)\)

Answer.

  1. \(\displaystyle m(5)=-1\)

  2. \(\displaystyle m(1)=6\)

  3. \(\displaystyle m(9)=-8\)

  4. \(\displaystyle m(3)=2\)

  5. \(\displaystyle m(7)=-5\)

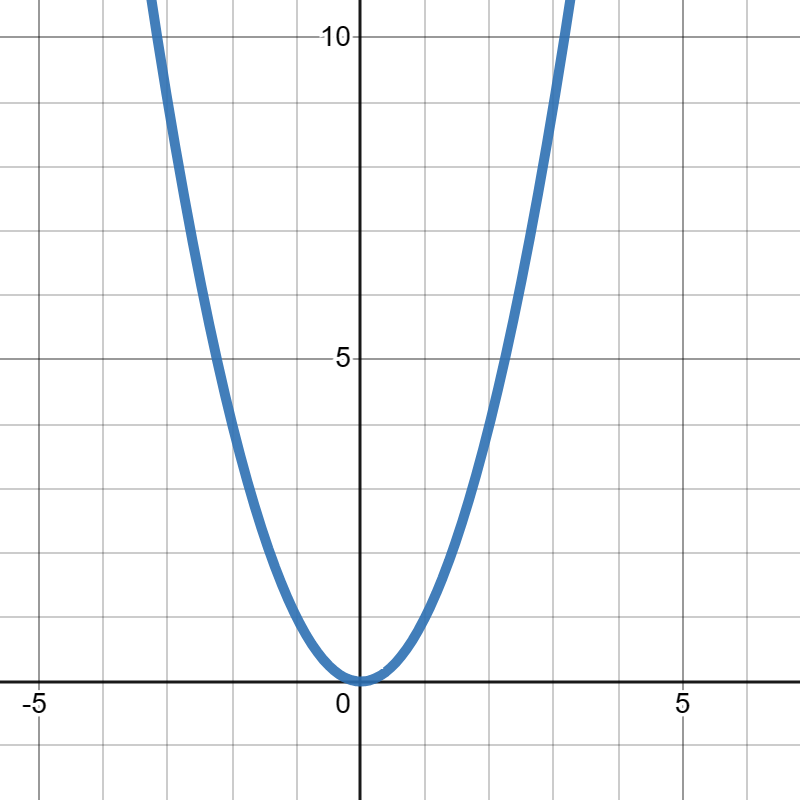
Exercise Group.

Suppose \(f(x)= 3x-3\) and \(g(x)\) is given in the graph below:

32.

Evaluate \(2f(2)-g(0)+1\text{.}\)

Answer.

\(2f(2)-g(0)+1=7\)

33.

Evaluate \(g(2)-(g(-2))^2\text{.}\)

Answer.

\(g(2)-(g(-2))^2=-12\)

34.

Evaluate \(5g(-1)+4f(3)\text{.}\)

Answer.

\(5g(-1)+4f(3)=-29\)

35.

Evaluate \(\frac{1}{2}f(5)-2f(0)\text{.}\)

Answer.

\(\frac{1}{2}f(5)-2f(0)=0\)

36.

Evaluate \((f(-2))^2-f(0)-(g(2))^3\text{.}\)

Answer.

\((f(-2))^2-f(0)-(g(2))^3=20\)

Exercise Group.

Suppose \(f(x)= x\) and \(g(x)\) is given in the table below:

Table 2.39.
x 1 2 3 4 5
g(x) 6 4 -1 7 0

37.

Evaluate \(2f(2)-g(1)+1\text{.}\)

Answer.

\(2f(2)-g(1)+1=-1\)

38.

Evaluate \(g(4)-(g(3))^3\text{.}\)

Answer.

\(g(4)-(g(3))^3=5\)

39.

Evaluate \(2g(1)-3f(5)\text{.}\)

Answer.

\(2g(1)-3f(5)=-3\)

40.

Evaluate \(\frac{1}{2}f(10)-3f(1)\text{.}\)

Answer.

\(\frac{1}{2}f(5)-3f(1)=2\)

41.

Evaluate \((f(-2))^2-g(2)-\frac{1}{3}g(5)\text{.}\)

Answer.

\((f(-2))^2-g(2)-\frac{1}{3}g(5)=0\)

Exercise Group.

Suppose \(g(x)\) is given in the graph below and \(h(x)\) is given in the table below:

Table 2.40.
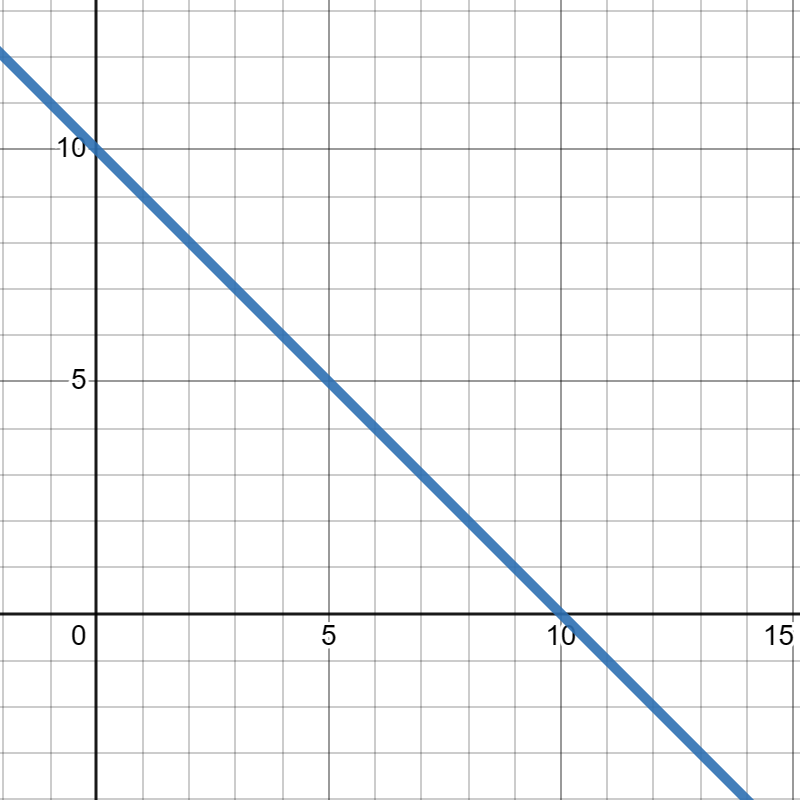
x 0 2 4 6 8
h(x) 2 3 9 1 4

42.

Evaluate \(4h(2)-g(0)+3\text{.}\)

Answer.

\(4h(2)-g(0)+3=5\)

43.

Evaluate \(g(4)-\frac{1}{2}(h(6))^3\text{.}\)

Answer.

\(g(4)-\frac{1}{2}(h(6))^3=5.5\)

44.

Evaluate \(2g(1)+4h(8)\text{.}\)

Answer.

\(2g(1)+4h(8)=34\)

45.

Evaluate \(\frac{1}{2}g(10)-3h(0)\text{.}\)

Answer.

\(\frac{1}{2}g(10)-3h(0)=-2\)

46.

Evaluate \((h(4))^2-6g(2)-\frac{1}{3}g(1)\text{.}\)

Answer.

\((h(4))^2-6g(2)-\frac{1}{3}g(1)=30\)

Exercise Group.

Suppose \(f(x)\) is given in the table below and \(g(x)=\frac{1}{2}x+2\text{:}\)

Table 2.41.
\(x\) \(f(x)\)
\(0\) \(2\)
\(1\) \(4\)
\(2\) \(6\)
\(3\) \(8\)
\(4\) \(10\)

47.

Evaluate \(3f(2)-g(2)+3\text{.}\)

Answer.

\(3f(2)-g(2)+3=18\)

48.

Evaluate \(g(10)-\frac{1}{4}(f(3))^2\text{.}\)

Answer.

\(g(10)-\frac{1}{4}(f(3))^2=-9\)

49.

Evaluate \(3g(1)+4f(4)\text{.}\)

Answer.

\(3g(1)+4f(4)=47.5\)

50.

Evaluate \(\frac{1}{5}g(6)-3f(0)\text{.}\)

Answer.

\(\frac{1}{5}g(6)-3f(0)=-5\)

51.

Evaluate \((f(1))^2-2g(2)-\frac{1}{3}g(-4)\text{.}\)

Answer.

\((f(1))^2-2g(2)-\frac{1}{3}g(-4)=10\)

Exercise Group.

Suppose \(f(x)= 4x-8\text{.}\)

52.

Solve \(f(x)=0\text{.}\)

Answer.

\(x=2\)

53.

Evaluate \(f(0)\text{.}\)

Answer.

\(f(0)=-8\)

54.

Evaluate \(f(2)\text{.}\)

Answer.

\(f(2)=0\)

55.

Solve \(f(x)=\frac{1}{2}\text{.}\)

Answer.

\(x=2.125\)

56.

Solve \(f(x)=10\text{.}\)

Answer.

\(x=4.5\)

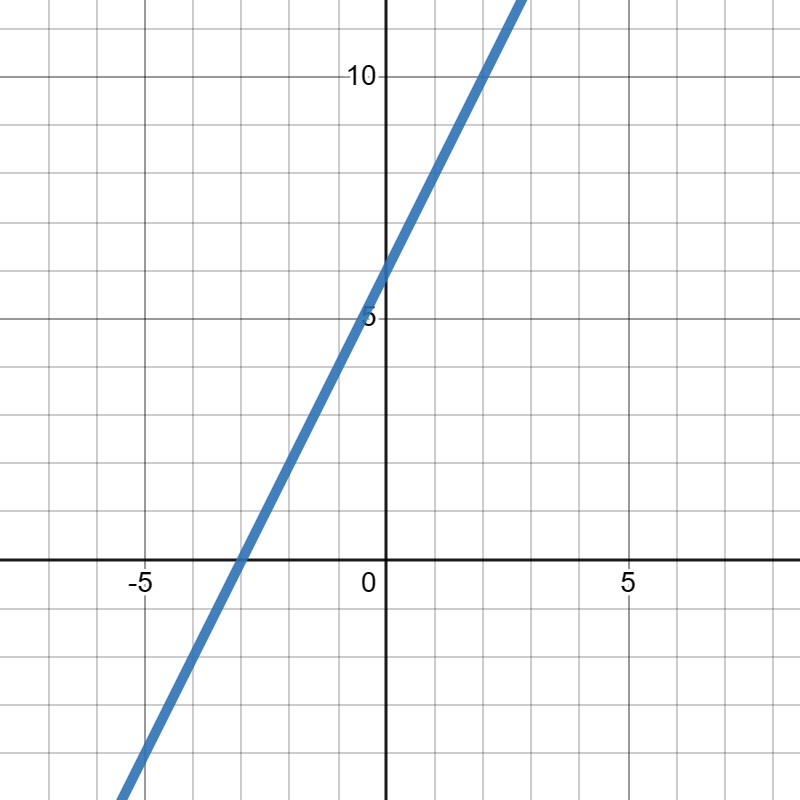
Exercise Group.

Suppose \(g(x)\) is given in the graph below.

57.

Solve \(g(x)=0\text{.}\)

Answer.

\(x=-3\)

58.

Evaluate \(g(0)\text{.}\)

Answer.

\(g(0)=6\)

59.

Evaluate \(g(1)\text{.}\)

Answer.

\(g(1)=8\)

60.

Solve \(g(x)=-2\text{.}\)

Answer.

\(x=-4\)

61.

Solve \(g(x)=4\text{.}\)

Answer.

\(x=-1\)

Exercise Group.

Suppose \(f(x)\) is given in the table below.

Table 2.42.
\(x\) \(f(x)\)
\(1\) \(3\)
\(2\) \(6\)
\(3\) \(9\)
\(4\) \(12\)
\(5\) \(15\)

62.

Solve \(f(x)=12\text{.}\)

Answer.

\(x=4\)

63.

Evaluate \(f(1)\text{.}\)

Answer.

\(f(1)=3\)

64.

Evaluate \(f(3)\text{.}\)

Answer.

\(f(3)=9\)

65.

Solve \(f(x)=6\text{.}\)

Answer.

\(x=2\)

66.

Solve \(f(x)=9\text{.}\)

Answer.

\(x=3\)

Exercise Group.

Suppose \(g(x)\) is given in the graph below.

67.

Solve \(g(x)=1\text{.}\)

Answer.

\(x=0,2\)

68.

Evaluate \(g(-1)\text{.}\)

Answer.

\(g(-1)=4\)

69.

Evaluate \(g(1)\text{.}\)

Answer.

\(g(1)=0\)

70.

Solve \(g(x)=6\text{.}\)

Answer.

\(x=6\)

71.

Solve \(g(x)=5\text{.}\)

Answer.

\(x=5\)

Exercise Group.

Suppose \(g(x)=(x-1)^2\) .

72.

Solve \(g(x)=9\text{.}\)

Answer.

\(x=-2,4\)

73.

Evaluate \(g(1)\text{.}\)

Answer.

\(g(0)=1\)

74.

Evaluate \(g(2)\text{.}\)

Answer.

\(g(2)=1\)

75.

Solve \(g(x)=16\text{.}\)

Answer.

\(x=-3,5\)

76.

Solve \(g(x)=0\text{.}\)

Answer.

\(x=1\)