Exercises 9.8 Practice Problems
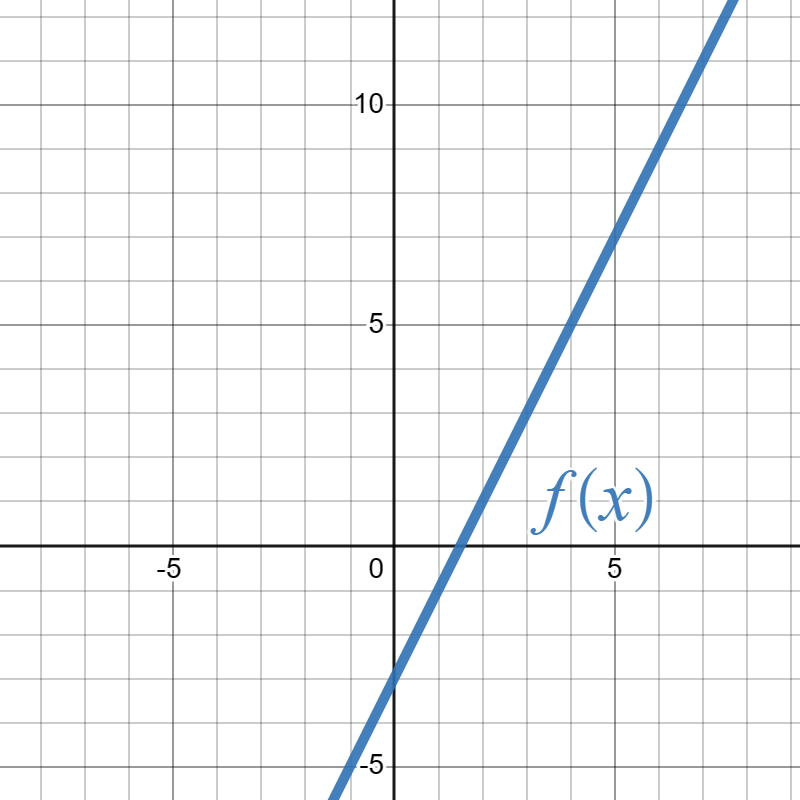
1.
Below is the graph of \(f(x)\text{.}\)
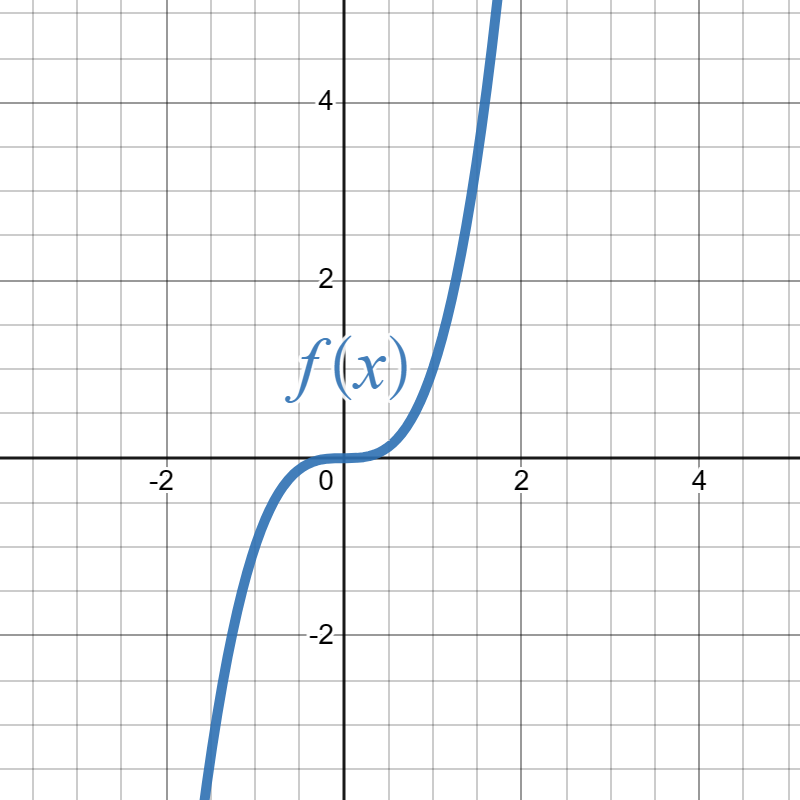
2.
Below is the graph of \(f(x)\text{.}\)
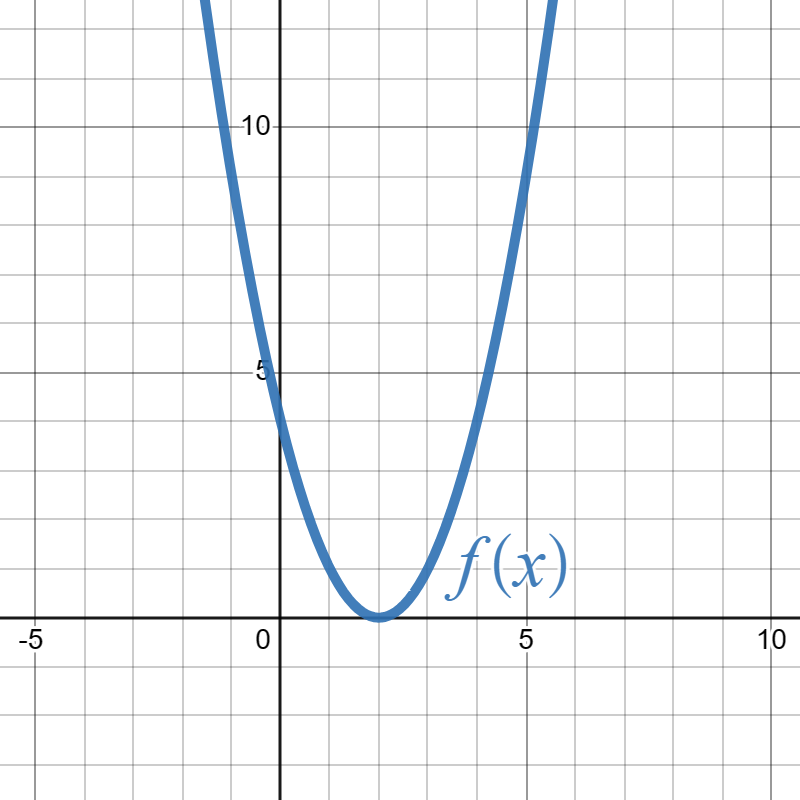
3.
Below is the graph of \(f(x)\text{.}\)
4.
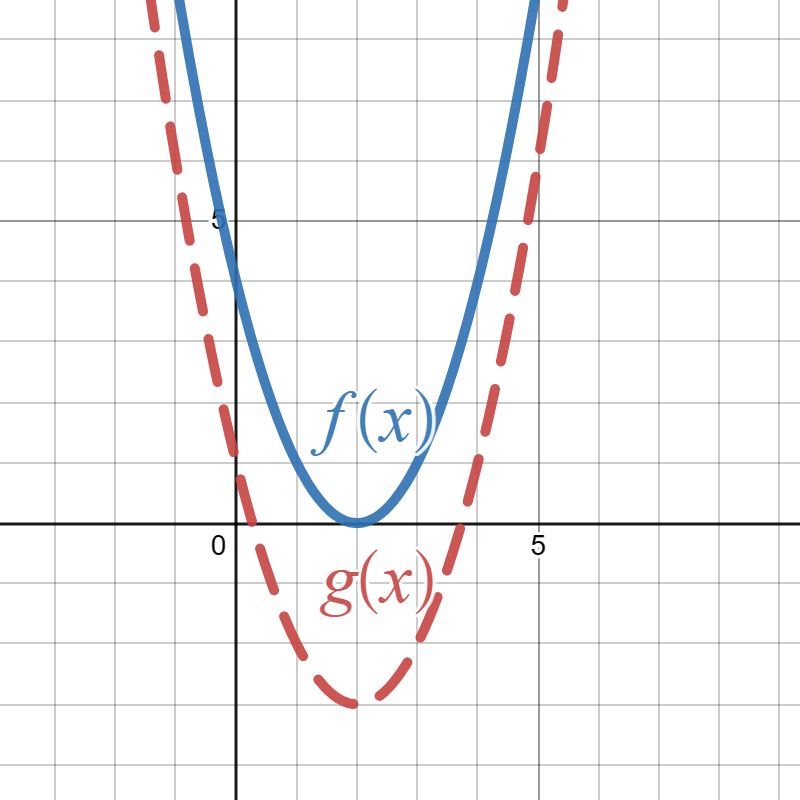
Suppose the solid blue graph below is the graph of \(f(x)\) and the red graph is the graph of \(g(x)\text{:}\)
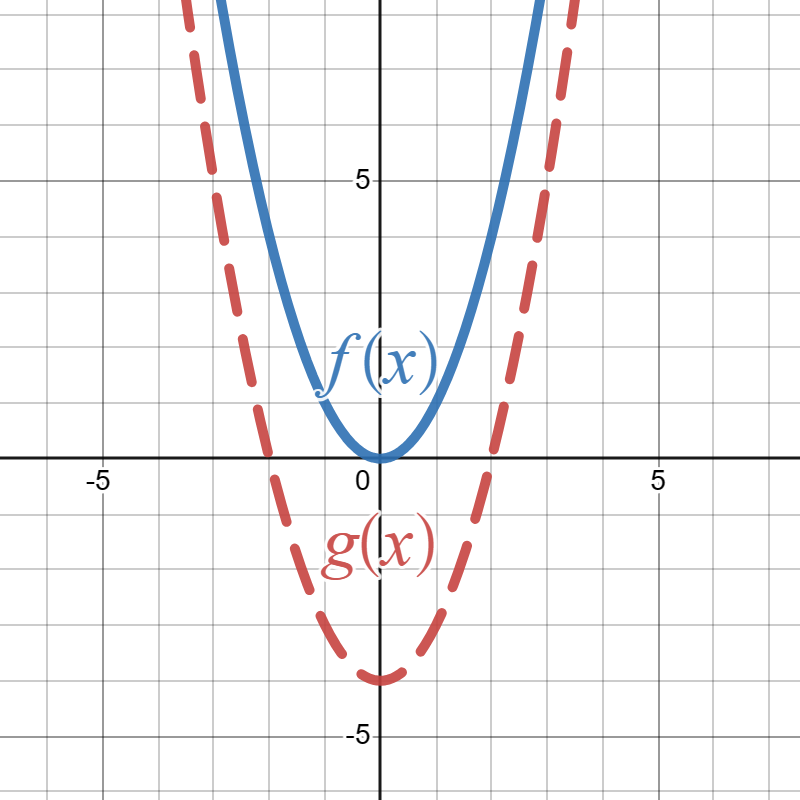
Write a formula for \(g(x)\) in terms of \(f(x)\text{.}\)
\(g(x)=f(x)-4\)
5.
Suppose the solid blue graph below is the graph of \(f(x)\) and the red graph is the graph of \(g(x)\text{:}\)
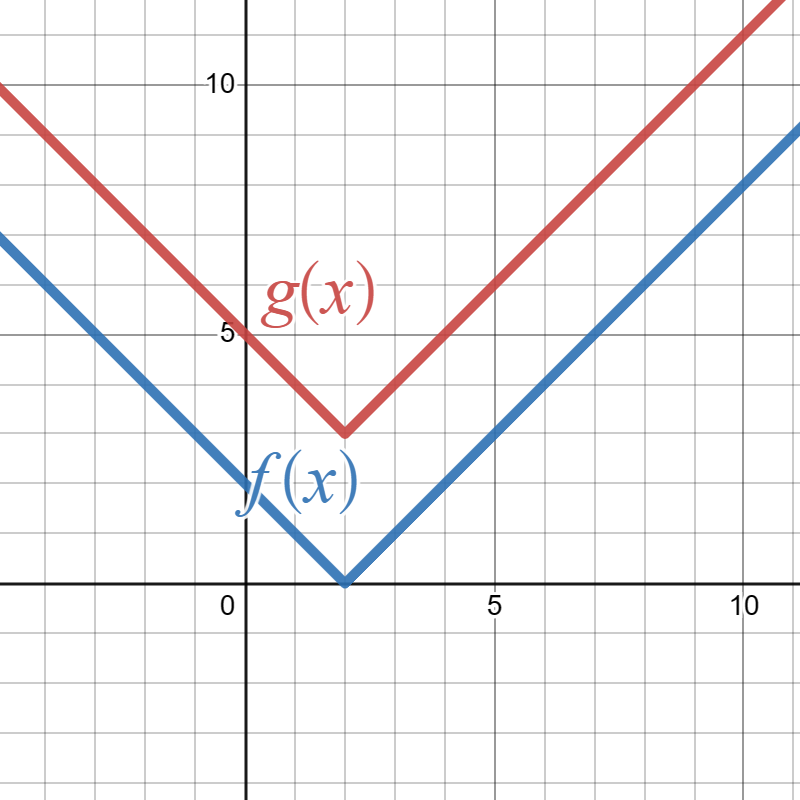
Write a formula for \(g(x)\) in terms of \(f(x)\text{.}\)
\(g(x)=f(x)+3\)
6.
Suppose \(f(x)=x^3-2x+1\) and \(g(x)\) is the same as \(f(x)\) but shifted down by \(3\text{.}\) Write a formula for \(g(x)\text{.}\)
\(g(x)=x^3-2x-2\)
7.
Suppose \(f(x)=3x^2\) and \(g(x)=3x^2-4\text{.}\) What transformations took \(f(x)\) to \(g(x)\text{?}\)
Shift down by \(4\text{.}\)
8.
Suppose \(f(x)=x^2-3\) and \(g(x)\) is the same as \(f(x)\) but shifted up by \(1\text{.}\) Write a formula for \(g(x)\text{.}\)
\(g(x)=x^2-2\)
9.
Suppose \(f(x)=3x+4\) and \(g(x)=3x+8\text{.}\) What transformations took \(f(x)\) to \(g(x)\text{?}\)
Shift up by \(4\text{.}\)